Optimal Merge Pattern Program In C
There are three methods that do the merging:- Suppose you are merging m lists with n elements each Algorithm 1:- Merge lists two at a time. Use merge sort like merge routine to merge as the lists are sorted. This is very simple to implement without any libraries. But takes time O(m^2.n) which is small enough if m is not large. Algorithm 2:- This is an improvement over 1. Where we always merge list which are the smallest two in the remaining list. Use a priority queue to do that and select smallest two list and merge them and add new list to queue.
C plus plus program for optimal merge. Cin>>n; cout. Already exists as an alternate of this question. Would you like to make it the primary and merge this question into it? So here we have 3 merge patterns available, out of which Case 3 is the optimal.
Do this till only 1 list is left which would be your answer. This technique is used in huffman coding and produces optimal merge pattern. This takes O(m.n.logm). Moreover for similar sized lists it can be made parallel as we can select a pair of list and merge in parallel.

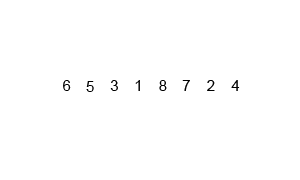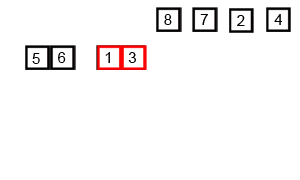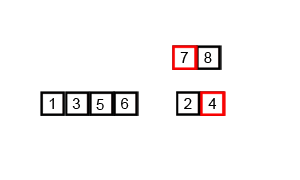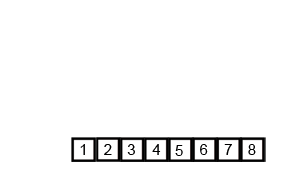
Assuming you have m processors then the algorithm can ideally run in O(n.logm) instead of O(m.n.logm) Algorithm 3:- This is most efficient algorithm where you maintain a priority queue for first elements of all lists and extract min to get new element also maintain index of the list min element belongs to so that you can add the next element from that list. This take O(s.logm) where s is total elements in all lists. Assumptions The following method works with any container like array, vector, list etc. I'm assuming that we are working with lists.
Let's assume that we have m sorted lists which we want to merge. Let n denotes the total number of elements in all lists. Vacuum pressure gauge psi.
Idea The first element in the resulting list has to be the smallest element in the set of all heads of the lists. The idea is quite simple. Just select the smallest head and move it from the original list to the result. You want to repeat that routine while there is at least one non empty list.

The crucial thing here is to select the smallest head fast. If m is small A linear scan through the heads is O(m) resulting in O(m. n) total time which is fine if m is a small constant.
Optimal Merge Pattern Program In C++
If m is not so small Then we can do better by using a priority queue, for example a heap. The invariant here is that the smallest element in the heap is always the smallest element from current heads.
Finding the minimum element is a heap is O(1), deleting the minimum is O(log m) if there are m elements in the heap, and inserting an element into the heap is also O(log m). In summary, for each of n elements, we insert it into the heap once and delete it from there also once. The total complexity with a heap is O(n log m) which is significantly faster that O(n. m) if m is not a small constant. Summary Which method is faster depends on how many lists we want to merge.
If m is small pick the linear scan, in the other case implement it with a priority queue. Sometimes it's hard to judge if the m is small or not and in that case some experiments will be helpful. The code for this could be similar to a pointer and count based merge sort, starting by creating a 'source' array of pointers and counts for each sequence, and allocating a second 'destination' array to merge the 'source' array of pointers and counts into. Each pass of this algorithm merges pairs of pointers and counts based on the sequences from the 'source' array into the 'destination' array, reducing the number of entries in the array by about 1/2.
Then pointers to the 'source' and 'destination' arrays are swapped, and the merge process repeated until an array of pointers and counts only has a single entry.
Merge sort runs in O (n log n) running time. It is very efficient sorting algorithm with near optimal number of comparison. Used for merge sort comes under the category of divide and conquer technique. An array of n elements is split around its center producing two smaller arrays. After these two arrays are sorted independently, they can be merged to produce the final sorted array.
Pattern Program In C
The process of splitting and merging can be carried recursively till there is only one element in the array. An array with 1 element is always sorted. ↓. ama Yes Yes Yes.I do have a doubt. Mergesort(a,i,mid); //left recursion mergesort(a,mid+1,j); //right recursion As per my understanding left recursion will execute until the condition is false and then the control moves over to the right recursion.
My question is at mergesort(a,mid+1,j),the new values of mid,low and high will be from main function? OR from the mergesort(a,i,mid)? And the same question applies here too: mergesort(a,mid+1,j); //right recursion merge(a,i,mid,mid+1,j); //merging of two sorted sub-arrays.